Pi’s (π) is one of the most well-known and intriguing mathematical constants. It represents the ratio of a circle’s circumference to its diameter and is an irrational number that has captivated mathematicians, scientists, and enthusiasts for centuries. This article delves into the fascinating world of Pi, exploring its history, mathematical significance, applications, and cultural impact.

The History of Pi
The journey of Pi dates back thousands of years. Ancient civilizations, including the Egyptians and Babylonians, recognized the importance of the ratio of a circle’s circumference to its diameter, although their approximations of Pi varied.
Early Approximations
- Babylonians: Around 1900 BCE, the Babylonians used a value of Pi as 3.125 (25/8), derived from their understanding of geometry and circle measurements.
- Egyptians: The Rhind Papyrus (circa 1650 BCE) suggests that the Egyptians approximated Pi to be around 3.1605 (256/81).
Greek Contributions
The Greeks made significant strides in understanding Pi. Archimedes of Syracuse (circa 287-212 BCE) is often credited with one of the first rigorous approaches to approximating Pi. By inscribing and circumscribing polygons around a circle, Archimedes calculated that Pi lies between 3.1408 and 3.1429.
Further Developments
Throughout history, mathematicians continued to refine the value of Pi:
- Liu Hui (circa 263 CE) from China improved the approximation of Pi using a 96-sided polygon, calculating Pi to be 3.1416.
- Zu Chongzhi (429-500 CE), another Chinese mathematician, calculated Pi to be between 3.1415926 and 3.1415927, remarkably accurate for his time.
- Madhava of Sangamagrama (circa 1400 CE) from India developed the Madhava-Leibniz series, a significant leap in approximating Pi using infinite series.
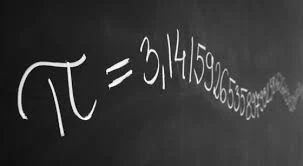
Mathematical Significance of Pi
Pi is an irrational number, meaning it cannot be expressed as a simple fraction and its decimal representation goes on infinitely without repeating. It is also a transcendental number, which means it is not a root of any non-zero polynomial equation with rational coefficients.
Properties of Pi
- Irrationality: Johann Lambert proved Pi’s irrationality in 1768, showing that it cannot be written as a fraction of two integers.
- Transcendence: In 1882, Ferdinand von Lindemann proved that Pi is transcendental, confirming that it cannot be the solution of any algebraic equation with rational coefficients.
Pi in Geometry
Pi is central to many geometric formulas, particularly those involving circles and spheres. Some key formulas include:
- Circumference of a circle: C=2πrC = 2\pi rC=2πr
- Area of a circle: A=πr2A = \pi r^2A=πr2
- Volume of a sphere: V=43πr3V = \frac{4}{3}\pi r^3V=34πr3
- Surface area of a sphere: A=4πr2A = 4\pi r^2A=4πr2

Pi in Calculus and Analysis
Pi appears in various integrals and infinite series in calculus. The Basel problem, solved by Leonhard Euler, involves the sum of the reciprocals of the squares of the natural numbers, leading to:
∑n=1∞1n2=π26\sum_{n=1}^\infty \frac{1}{n^2} = \frac{\pi^2}{6}∑n=1∞n21=6π2
Pi also emerges in Fourier series, which are used to express functions as the sum of sine and cosine functions.
Applications of Pi
Pi is not just a theoretical concept; it has practical applications across numerous fields, including engineering, physics, and computer science.
Engineering
In engineering, Pi is essential for designing and analyzing structures and systems involving circular and spherical shapes. For instance, Pi is used in calculating the stress and strain on cylindrical components, determining the flow of fluids through pipes, and designing gears and turbines.
Physics
Pi plays a crucial role in various physical equations and principles. It appears in formulas for wave mechanics, quantum mechanics, and electromagnetism. For example:
- Wave mechanics: The wave number kkk of a sinusoidal wave is given by k=2πλk = \frac{2\pi}{\lambda}k=λ2π, where λ\lambdaλ is the wavelength.
- Quantum mechanics: The Schrödinger equation, which describes the behavior of quantum particles, involves Pi in its formulation.
- Electromagnetism: Maxwell’s equations, which describe the behavior of electric and magnetic fields, include Pi in their integral forms.
Computer Science
In computer science, Pi is used in algorithms for numerical simulations, computer graphics, and cryptography. Calculating the digits of Pi has been a benchmark for testing the performance of supercomputers and algorithms. The quest to compute more digits of Pi has driven advancements in computational methods and hardware.
Cultural Impact of Pi
Pi has transcended the realm of mathematics and science, becoming a cultural icon with its own dedicated day and presence in popular culture.
Pi Day
Pi Day is celebrated on March 14th (3/14) each year, aligning with the first three digits of Pi. The celebration includes various activities, such as reciting the digits of Pi, pie-eating contests, and educational events to promote mathematics and science.
Pi in Literature and Media
Pi has made appearances in literature, films, and music. In the novel “Life of Pi” by Yann Martel, the protagonist’s name, Piscine Molitor Patel, is shortened to Pi, symbolizing his quest for meaning and survival. The film adaptation further popularized the association.
Pi Memorization
Memorizing the digits of Pi, known as “piphilology,” has become a popular challenge. Individuals and groups attempt to recite as many digits as possible, with current world records reaching tens of thousands of digits.
The Infinite Nature of Pi
One of the most fascinating aspects of Pi is its infinite and non-repeating decimal representation. This infinite nature has led to numerous studies and explorations, including:
Patterns and Randomness
While Pi’s digits are non-repeating, they have been extensively analyzed for patterns and randomness. Studies have shown that Pi’s digits are statistically random, with no discernible patterns over millions of digits.
Computational Advances
The quest to calculate more digits of Pi has driven significant advancements in computational methods. From Archimedes’ polygons to modern algorithms like the Gauss-Legendre algorithm and the Chudnovsky algorithm, each method has pushed the boundaries of precision and computation.
Mathematical Curiosities
Pi’s infinite nature has led to various mathematical curiosities and paradoxes. For example, Buffon’s Needle is a probability problem that estimates the value of Pi using random needle drops on a lined surface.

The Importance of Pi: A Deeper Exploration
Pi (π) is not just a mathematical constant; it is a fundamental element that underpins much of our understanding of the world. Its importance extends beyond the confines of geometry and mathematics into various fields such as physics, engineering, computer science, and even finance. Let’s explore the significance of Pi in more detail and understand why it holds such a pivotal place in both theoretical and applied sciences.
Pi in Geometry and Mathematics
Pi’s most straightforward application is in geometry, where it is essential for calculations involving circles and other curved shapes. Its importance, however, extends to more advanced mathematical theories and practices.
Fundamental Geometric Calculations
Pi is crucial in determining the circumference and area of circles, which are foundational concepts in geometry.
- Circumference of a circle: C=2πrC = 2\pi rC=2πr, where rrr is the radius.
- Area of a circle: A=πr2A = \pi r^2A=πr2.
These formulas are not only used in theoretical mathematics but also in practical applications like designing wheels, gears, and other circular objects.
Trigonometry and Fourier Series
Pi plays a critical role in trigonometry, especially in the properties of sine and cosine functions, which are periodic functions with a period of 2π2\pi2π. This periodic nature is essential for analyzing waves and oscillations, which appear in various physical phenomena, from sound waves to electromagnetic waves.
In the Fourier series, Pi is used to decompose complex periodic functions into sums of simpler sine and cosine functions. This decomposition is fundamental in signal processing, enabling the analysis and synthesis of signals.
Pi in Physics
Pi’s importance in physics cannot be overstated. It appears in numerous physical laws and formulas, often relating to circular or spherical shapes, waves, and oscillations.
Waves and Oscillations
In wave mechanics, Pi appears in the relationship between the wavelength (λ\lambdaλ) and the wave number (kkk):
k=2πλk = \frac{2\pi}{\lambda}k=λ2π
This relationship is crucial for understanding wave propagation, resonance, and the behavior of different types of waves, including light and sound.
Quantum Mechanics
In quantum mechanics, Pi is integral to the Schrödinger equation, which describes how the quantum state of a physical system changes over time. Pi appears in the normalization of wave functions, ensuring that the total probability of finding a particle is one.
Relativity and Cosmology
Pi is also present in Einstein’s field equations of general relativity, which describe the gravitational interactions between masses. These equations involve complex integrations over curved spacetime, where Pi is a constant that appears naturally.
In cosmology, Pi helps describe the geometry of the universe. For instance, the curvature of space can be described using spherical geometry, where Pi plays a fundamental role.
Pi in Engineering
Engineers use Pi extensively in designing and analyzing systems and structures, particularly those involving circular or spherical shapes.
Structural Engineering
In structural engineering, Pi is used to calculate properties of cylindrical and spherical structures. For example, the stress and strain in pipes, tanks, and pressure vessels are determined using formulas involving Pi.
Mechanical Engineering
Mechanical engineers use Pi in designing gears, bearings, and turbines. The efficiency and performance of these components depend on precise calculations of their circular dimensions.
Electrical Engineering
In electrical engineering, Pi appears in the analysis of AC circuits, which involve sinusoidal voltages and currents. The relationship between the frequency of these signals and their angular frequency (ω\omegaω) is given by:
ω=2πf\omega = 2\pi fω=2πf
where fff is the frequency. This relationship is fundamental for designing and analyzing circuits, filters, and communication systems.
Pi in Computer Science
Pi has significant applications in computer science, particularly in algorithms, simulations, and numerical analysis.
Computational Algorithms
Calculating the digits of Pi has been a benchmark for testing the performance and accuracy of algorithms and computer hardware. Efficient algorithms for computing Pi, such as the Gauss-Legendre algorithm and the Chudnovsky algorithm, have advanced the field of numerical analysis.
Simulations and Modeling
Pi is used in simulations that involve circular or spherical geometries, such as modeling the motion of planets, simulating fluid dynamics, and analyzing heat transfer in spherical objects. These simulations are crucial for understanding complex physical systems and making predictions.
Cryptography
In cryptography, Pi’s seemingly random sequence of digits is used to generate random numbers, which are essential for secure encryption algorithms. Although Pi itself is not used directly, the principles of randomness and unpredictability are inspired by its properties.
Pi in Economics and Finance
Pi even finds applications in economics and finance, where it helps model cyclical behaviors and optimize investment strategies.
Cyclical Patterns
Economic cycles, such as business cycles and seasonal variations, can be modeled using sine and cosine functions, which are inherently related to Pi. Understanding these cycles is crucial for making informed economic decisions and predictions.
Optimization
In finance, Pi is used in optimization algorithms that aim to maximize returns or minimize risks. These algorithms often involve mathematical functions that require precise calculations, where Pi plays a role.
Cultural and Philosophical Significance
Beyond its scientific and practical applications, Pi has a profound cultural and philosophical significance.
Pi in Popular Culture
Pi has permeated popular culture, appearing in movies, books, and art. “Life of Pi,” a novel by Yann Martel, uses Pi both as a name for the protagonist and as a metaphor for the infinite and the unknowable. Movies like “Pi” by Darren Aronofsky explore the theme of obsession with mathematical patterns and the mysteries of the universe.
Philosophical Implications
Pi’s infinite and non-repeating nature has philosophical implications, symbolizing the concept of infinity and the limits of human understanding. It serves as a reminder of the complexity and beauty of the universe, inspiring awe and curiosity.
Educational Impact
Pi Day, celebrated on March 14th (3/14), has become an educational event promoting mathematics and science. Activities on Pi Day aim to engage students and the public in appreciating the significance of mathematics in everyday life.
If you want to check the mystery of angel number 47 then click on the link below:
Angel Number 47: A Message from the Divine
Conclusion
Pi is more than just a mathematical constant; it is a symbol of the infinite, the mysterious, and the interconnectedness of mathematics, science, and culture. From its ancient approximations to its modern-day applications, Pi continues to captivate and inspire. Whether you are a mathematician, a scientist, or simply a curious mind, exploring the world of Pi offers endless opportunities for discovery and wonder. As we continue to push the boundaries of knowledge and computation, Pi remains a testament to the beauty and complexity of the universe.







Be First to Comment